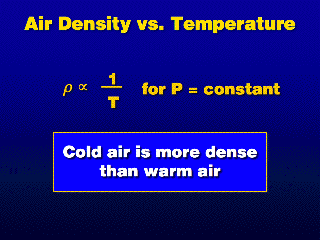
 The Ideal Gas Law is used to derive the relation that warm air is less dense
than cold air at the same pressure.
The Ideal Gas Law is used to derive the relation that warm air is less dense
than cold air at the same pressure. The Ideal Gas Law is used to derive the relation that warm air is less dense
than cold air at the same pressure.
The Ideal Gas Law is used to derive the relation that warm air is less dense
than cold air at the same pressure.
As the air temperature increases ("warm air"), the density
decreases at constant pressure. In the atmosphere, this is saying that when
we compare the density of two air samples, they have to be at the same altitude,
since that will provide us with the constant pressure assumption. This works
out well for air parcels, because the parcel pressure inside is always the
same as the environmental pressure outside.
 For air parcels, we look at the temperature inside vs. the temperature outside
to get a density difference that results in positive or negative buoyancy.
Air parcels that are colder than the surrounding air are negatively buoyant
and tend to sink (there is a net buoyancy force downward; air parcels that are
warmer than the surrounding air are positively bouyant and tend to rise (net
buoyancy force upward).
For air parcels, we look at the temperature inside vs. the temperature outside
to get a density difference that results in positive or negative buoyancy.
Air parcels that are colder than the surrounding air are negatively buoyant
and tend to sink (there is a net buoyancy force downward; air parcels that are
warmer than the surrounding air are positively bouyant and tend to rise (net
buoyancy force upward).
Most of the atmosphere is not going up or down, so there is neutral buoyancy--the air inside some air parcel is the same temperature (and density) as the air outside. There is no net buoyancy force, since in this case, the upward pressure buoyancy force is equal to the gravitational force acting downward on the air mass inside the parcel (this also is the case for hydrostatic equilibrium).
Okay, so how are we going to get moving parcels if we normally start with the
parcel temperature and density being the same as the surrounding environment?
We move the parcel around in the atmosphere, and if the
parcel temperature varies differently than the surrounding atmosphere, then
it will end up with a different temperature than the atmosphere, and either
positively or negatively buoyant.